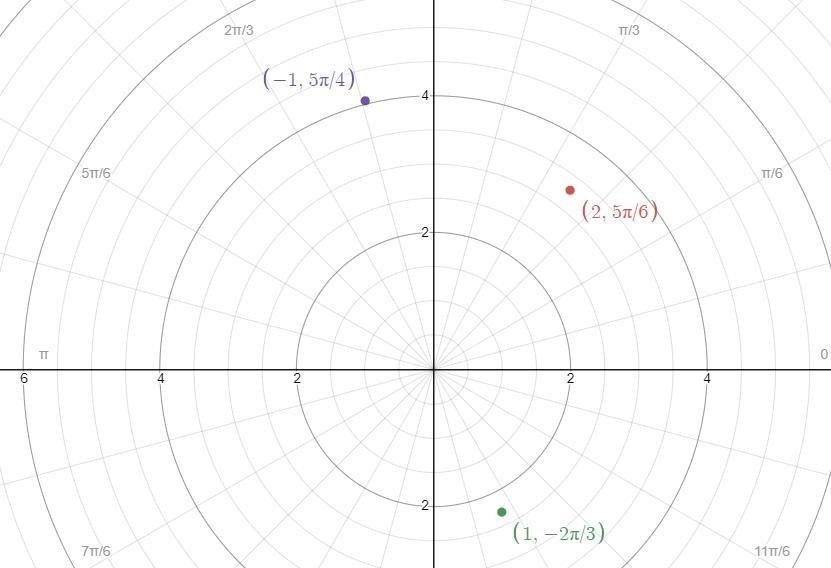
Plot the point whose polar coordinates are given. Then find two other pairs of polar coordinates of this point, one with r > 0, and one with r < 0.
(a) (2, 5pi/6)
(b) (1, -2pi/3)
(c) (-1, 5pi/4)

Answer:

The other pairs are:

[tex](a)\ (2, \frac{5\pi}{6}) \to[/tex]  [tex](2, \frac{17\pi}{6})[/tex] and [tex](-2, \frac{23\pi}{6})[/tex]

[tex](b)\ (1, -\frac{2\pi}{3}) \to[/tex] [tex](1, \frac{4\pi}{3})[/tex] and [tex](-1, \frac{7\pi}{3})[/tex]

[tex](c)\ (-1, \frac{5\pi}{4}) \to[/tex] [tex](-1, \frac{3\pi}{4} )[/tex] and [tex](1, \frac{7\pi}{4})[/tex]

See attachment for plots

Step-by-step explanation:

Given

[tex](a)\ (2, \frac{5\pi}{6})[/tex]

[tex](b)\ (1, -\frac{2\pi}{3})[/tex]

[tex](c)\ (-1, \frac{5\pi}{4})[/tex]

Solving (a): Plot a, b and c

See attachment for plots

Solving (b): Find other pairs for [tex]r > 0[/tex] and [tex]r < 0[/tex]

The general rule is that:

The other points can be derived using

[tex](r, \theta) = (r, \theta + 2n\pi)[/tex]

and

[tex](r, \theta) = (-r, \theta + (2n + 1)\pi)[/tex]

Let [tex]n =1[/tex] ---- You can assume any value of n

So, we have:

[tex](r, \theta) = (r, \theta + 2n\pi)[/tex]

[tex](r, \theta) = (r, \theta + 2*1*\pi)[/tex]

[tex](r, \theta) = (r, \theta + 2\pi)[/tex]

[tex](r, \theta) = (-r, \theta + (2n + 1)\pi)[/tex]

[tex](r, \theta) = (-r, \theta + (2*1 + 1)\pi)[/tex]

[tex](r, \theta) = (-r, \theta + (2 + 1)\pi)[/tex]

[tex](r, \theta) = (-r, \theta + 3\pi)[/tex]

[tex](a)\ (2, \frac{5\pi}{6})[/tex]

[tex]r = 2\ \ \ \ \theta = \frac{5\pi}{6}[/tex]      

So, the pairs are:

[tex](r, \theta) = (r, \theta + 2\pi)[/tex]

[tex](2, \frac{5\pi}{6}) = (2, \frac{5\pi}{6} + 2\pi)[/tex]

Take LCM

[tex](2, \frac{5\pi}{6}) = (2, \frac{5\pi+12\pi}{6})[/tex]

[tex](2, \frac{5\pi}{6}) = (2, \frac{17\pi}{6})[/tex]

And

[tex](r, \theta) = (-r, \theta + 3\pi)[/tex]

[tex](2, \frac{5\pi}{6}) = (-2, \frac{5\pi}{6} + 3\pi)[/tex]

Take LCM

[tex](2, \frac{5\pi}{6}) = (-2, \frac{5\pi+18\pi}{6})[/tex]

[tex](2, \frac{5\pi}{6}) = (-2, \frac{23\pi}{6})[/tex]

The other pairs are:

[tex](2, \frac{17\pi}{6})[/tex] and [tex](-2, \frac{23\pi}{6})[/tex]

[tex](b)\ (1, -\frac{2\pi}{3})[/tex]

[tex]r = 1\ \ \ \theta = -\frac{2\pi}{3}[/tex]      

So, the pairs are:

[tex](r, \theta) = (r, \theta + 2\pi)[/tex]

[tex](1, -\frac{2\pi}{3}) = (1, -\frac{2\pi}{3} + 2\pi)[/tex]

Take LCM

[tex](1, -\frac{2\pi}{3}) = (1, \frac{-2\pi+6\pi}{3})[/tex]

[tex](1, -\frac{2\pi}{3}) = (1, \frac{4\pi}{3})[/tex]

And

[tex](r, \theta) = (-r, \theta + 3\pi)[/tex]

[tex](1, -\frac{2\pi}{3}) = (-1, -\frac{2\pi}{3} + 3\pi)[/tex]

Take LCM

[tex](1, -\frac{2\pi}{3}) = (-1, \frac{-2\pi+9\pi}{3})[/tex]

[tex](1, -\frac{2\pi}{3}) = (-1, \frac{7\pi}{3})[/tex]

The other pairs are:

[tex](1, \frac{4\pi}{3})[/tex] and [tex](-1, \frac{7\pi}{3})[/tex]

[tex](c)\ (-1, \frac{5\pi}{4})[/tex]

[tex]r = -1 \ \ \ \ \theta = \frac{-5\pi}{4}[/tex]

So, the pairs are

[tex](r, \theta) = (r, \theta + 2\pi)[/tex]

[tex](-1, \frac{-5\pi}{4}) = (-1, \frac{-5\pi}{4} + 2\pi)[/tex]

Take LCM

[tex](-1, \frac{-5\pi}{4}) = (-1, \frac{-5\pi+8\pi}{4} )[/tex]

[tex](-1, \frac{-5\pi}{4}) = (-1, \frac{3\pi}{4} )[/tex]

And

[tex](r, \theta) = (-r, \theta + 3\pi)[/tex]

[tex](-1, \frac{-5\pi}{4}) = (-(-1), \frac{-5\pi}{4}+ 3\pi)[/tex]

Take LCM

[tex](-1, \frac{-5\pi}{4}) = (1, \frac{-5\pi+12\pi}{4})[/tex]

[tex](-1, \frac{-5\pi}{4}) = (1, \frac{7\pi}{4})[/tex]

So, the other pairs are:

[tex](-1, \frac{3\pi}{4} )[/tex] and [tex](1, \frac{7\pi}{4})[/tex]

Kenny loves to drink milk. He drinks 20 quarts of milk in 5 weeks. How many quarts of milk will Kenny drink in 12 weeks?

can someone please help me on this question?

Graph the function n(x) = |x6|.
+Move Ray
-6
-4
-2
104
9
4
2
0
-2
Undo
2
4
Redo
6
x Reset
8
10

PLEASE HELP, ASAP. I WILL MARK YOU BRAINLIEST‼️

y≥ −4x+10
Hello, I’m having trouble the the following question above. Can you help me please?
The question asks to name the A and B points
A: ( , )
B: ( , )

what is the slope of the table below? 3/8 15/12 9/10 0/7

Please help giving brainliest please

Find the exact value of the expression

A box office sold 147,523 tickets for an auto race.
Of this number, 68,724 tickets were for adults.
The rest were children's tickets.

How many children's tickets were sold?

78,709

81,799

121,201

If you save up $100 every 2 weeks, how much money would you have in a year ?

What is the distance, rounded to the nearest tenth, between the points (-2,4) and (6,-4)?

​

In ΔABC, the measure of ∠C=90°, AB = 86 feet, and CA = 61 feet. Find the measure of ∠A to the nearest degree.

Bernita and Rosalee are comparing their heights. Bernita is 1.63
meters tall. Rosalee is 19 centimeters shorter than Bernita.
What is Rosalee's height in centimeters?

What is the gravitational potential energy of a 55-kg person that has climber a 4,000 meter tall mountain?

Bonus: if one snickers bar is 1,100,000 joules of energy, how many snickers bars would provide enough fuel to climb the mountain ?

Solve for l:
[tex]v = \frac{ \sqrt{l + t} }{2 \sqrt{l} } [/tex]
.....​

Worked Example:
Select all the expressions that have the same value as 4 1/2% of 50.
A. 0.045x50
B. 4.5%x50
C. 43%x50
D. 4.5x50
E. 0.45x50
F. 412x50

What is the range of the function x is 0 and y is 40

evaluate the following polynomial when x = - 3
+6x² – 3x+5
2x

Kind of stuck any tips would also help!!

express each number as a product of two fractions 1/5

Use the figures to complete the statements proving the converse of the Pythagorean theorem.

Drag and drop a phrase, value, or equation into the box to correctly complete the proof.

Put responses in the correct input to answer the question. Select a response, navigate to the desired input and insert the response. Responses can be selected and inserted using the space bar, enter key, left mouse button or touchpad. Responses can also be moved by dragging with a mouse.
To prove the converse of the Pythagorean theorem, we can define a right triangle, Response area, with sides a, b, and x. Then, we will show that if ​△ABC​ is a triangle with sides a, b, and c where a² + b² = c², then it is congruent to △DEF and therefore a right triangle.

By the Pythagorean theorem, because ​△DEF​ is a right triangle, a² + b² = x².

If ​​a² + b² = x² and a² + b² = c² ​​, then c² = x². Further, since sides of triangles are positive, then we can conclude that ​c = x​. Thus, the two triangles have congruent sides and are congruent.

If ​△ABC​ is congruent to a right triangle, then it must also be a right triangle.

please help me to solve this​

Find the sum: −11−7−3+1+⋯+225

Which is 3 logx + 4 log(x-2) written as a single logarithm?
logx (x - 2)
logx(x-2)
b. 12 logx(x - 2)
d. 12 logx(x-2)

find the volume of each figure

Write two numbers that are opposites and more than 6 units away from 0.

Someone please help with this!

Which graph represents an exponential function?

A reporter for a student newspaper is writing an article on the cost of off-campus housing. A sample was selected of 10 one-bedroom units within a half-mile of campus and the rents paid. The sample mean is $550 and the sample standard deviation is $60.05. Provide a 95% confidence interval estimate of the mean rent per month for the population of one-bedroom units within a half-mile of campus. Assume that population is normally distributed.

Which list classifications for the figure